How To Draw A Tangent Line On A Graph Matlab

Quick Overview

• To find the equation of a line y'all demand a point and a slope.
• The slope of the tangent line is the value of the derivative at the point of tangency.
• The normal line is a line that is perpendicular to the tangent line and passes through the point of tangency.

Examples

Instance 1

Suppose $$f(10) = x^3$$. Observe the equation of the tangent line at the point where $$ten = 2$$.

Pace 1

Discover the point of tangency.

Since $$10=two$$, nosotros evaluate $$f(2)$$.

$$ f(ii) = 2^3 = viii $$

The betoken is $$(two,8)$$.

Step 2

Find the value of the derivative at $$x = ii$$.

$$ f'(10) = 3x^2\longrightarrow f'(2) = 3(2^2) = 12 $$

The the gradient of the tangent line is $$m = 12$$.

Step 3

Notice the point-slope class of the line with slope $$m = 12$$ through the signal $$(2,viii)$$.

$$ \begin{align*} y - y_1 & = m(10-x_1)\\[6pt] y - viii & = 12(x-2) \end{align*} $$

Answer

$$y - eight = 12(x-2)$$

For reference, here is the graph of the function and the tangent line we just found.

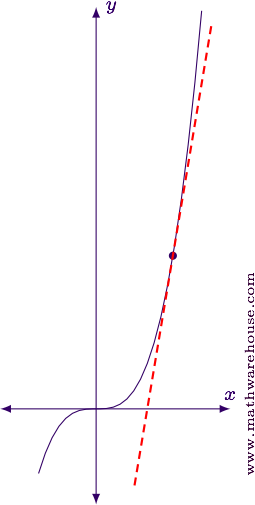
Case ii

Suppose $$f(x) = x^2 - x$$. Find the equation of the tangent line with slope $$m = -iii$$.

Footstep 1

Find the derivative.

$$ f'(x) = 2x -1 $$

Stride ii

Find the $$10$$-value where $$f'(x)$$ equals the slope.

$$ \brainstorm{align*} f'(x) & = 2x -ane\\[6pt] -3 & = 2x -1\\[6pt] -2 & = 2x\\[6pt] ten & = -1 \end{align*} $$

Step three

Observe the bespeak on the part where $$x = -1$$.

$$ f(-one) = (-1)^2 - (-one) = 1 + 1 = ii $$

The point is $$(-1, 2)$$.

Footstep 4

Discover the equation of the line through the bespeak $$(-1,2)$$ with slope $$m=-3$$.

$$ \begin{align*} y -y_1 & = 1000(10-x_1)\\[6pt] y - 2 & = -3(ten - (-1))\\[6pt] y - two & = -3(10+1) \end{align*} $$

Answer

$$ y - 2 = -iii(x+1) $$

For reference, here's the graph of the function and the tangent line we only found.

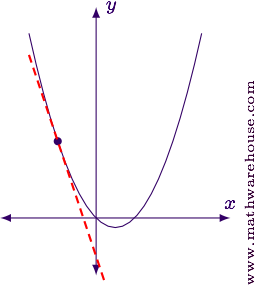
Tangent Lines to Implicit Curves

The procedure doesn't modify when working with implicitly defined curves.

Instance three

Suppose $$x^2 + y^2 = sixteen$$. Find the equation of the tangent line at $$x = 2$$ for $$y>0$$.

Stride 1

Observe the $$y$$-value of the signal of tangency.

$$ \brainstorm{align*} \blue{ten^2} + y^2 & = 16\\[6pt] \blue{2^ii} + y^2 & = sixteen\\[6pt] \blue{4} + y^two & = 16\\[6pt] y^2 & = 12\\[6pt] y & = \pm\sqrt{12}\\[6pt] y & = \pm\sqrt{4\cdot three}\\[6pt] y & = \pm2\sqrt 3 \end{align*} $$

Since the problem states we are interested in $$y>0$$, we use $$y = ii\sqrt 3$$.

The signal of tangency is $$(ii, 2\sqrt 3)$$.

Step 2

Observe the equation for $$\frac{dy}{dx}$$.

Since the equation is implicitly defined, we apply implicit differentiation.

$$ \begin{align*} 2x + 2y\,\frac{dy}{dx} & = 0\\[6pt] 2y\,\frac{dy}{dx} & = -2x\\[6pt] \frac{dy}{dx} & = -\frac{2x}{2y}\\[6pt] \frac{dy}{dx} & = -\frac x y \terminate{align*} $$

Stride iii

Find the slope of the tangent line at the point of tangency.

At the point $$(2,2\sqrt 3)$$, the slope of the tangent line is

$$ \begin{align*} \frac{dy}{dx}\bigg|_{(\blueish{2},\crimson{2\sqrt 3})} & = -\frac {\blue two} {\red{2\sqrt 3}}\\[6pt] & = -\frac one {\sqrt 3}\\[6pt] & = -\frac 1 {\sqrt 3}\cdot \blue{\frac{\sqrt three}{\sqrt iii}}\\[6pt] & = -\frac{\sqrt 3} 3 \finish{align*} $$

The gradient of the tangent line is $$chiliad = -\frac{\sqrt 3} 3$$.

Pace four

Find the equation of the tangent line through $$(2,2\sqrt 3)$$ with a slope of $$one thousand=-\frac{\sqrt 3} three$$.

At the indicate $$(ii,2\sqrt 3)$$, the slope of the tangent line is

$$ \brainstorm{align*} y - y_1 & = m(10-x_1)\\[6pt] y - two\sqrt 3 & = -\frac{\sqrt three} 3(10-2) \end{align*} $$

Answer

The equation of the tangent line is $$y - 2\sqrt iii = -\frac{\sqrt three} 3(x-2)$$

For reference, the graph of the curve and the tangent line we plant is shown below.

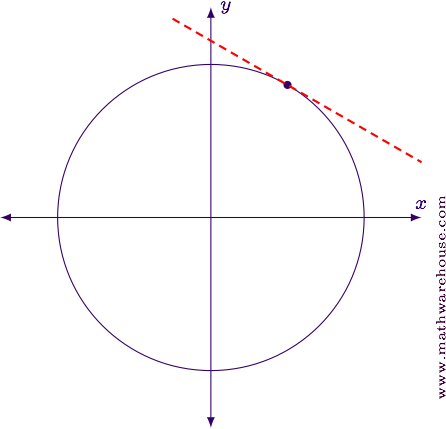
Normal Lines

Suppose we have a a tangent line to a function. The function and the tangent line intersect at the point of tangency. The line through that same point that is perpendicular to the tangent line is called a normal line.

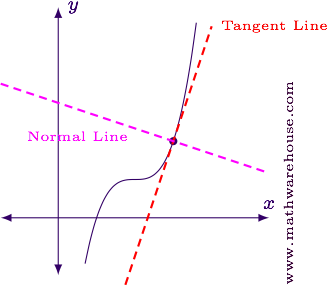
Recall that when two lines are perpendicular, their slopes are negative reciprocals. Since the slope of the tangent line is $$g = f'(ten)$$, the gradient of the normal line is $$yard = -\frac i {f'(10)}$$.

Instance 4

Suppose $$f(x) = \cos x$$. Detect the equation of the line that is normal to the function at $$x = \frac \pi 6$$.

Step 1

Observe the point on the function.

$$ f\left(\frac \pi six\right) = \cos \frac \pi vi = \frac{\sqrt 3} ii $$

The point is $$\left(\frac \pi 6, \frac{\sqrt 3} two\right)$$.

Step 2

Observe the value of the derivative at $$x = \frac \pi 6$$.

$$ f'(x) = -\sin x\longrightarrow f'\left(\frac \pi vi\right) = -\sin\frac\pi 6 = -\frac 1 2 $$

The gradient of the tangent line is $$m = -\frac 1 2$$. Since we are looking for the line that is perpendicular to the tangent line, nosotros want to use $$one thousand = 2$$.

Step 3

Find the equation of the line through the point $$\left(\frac \pi 6, \frac{\sqrt 3} 2\right)$$ with a slope of $$m =two$$.

$$ \begin{align*} y -y_1 & = m(x-x_1)\\[6pt] y - \frac{\sqrt 3} 2 & = 2\left(10 - \frac \pi 6\right) \end{align*} $$

Answer

The line normal to the function at $$10 = \frac \pi 6$$ is $$y - \frac{\sqrt 3} 2 = 2\left(x - \frac \pi 6\right)$$.

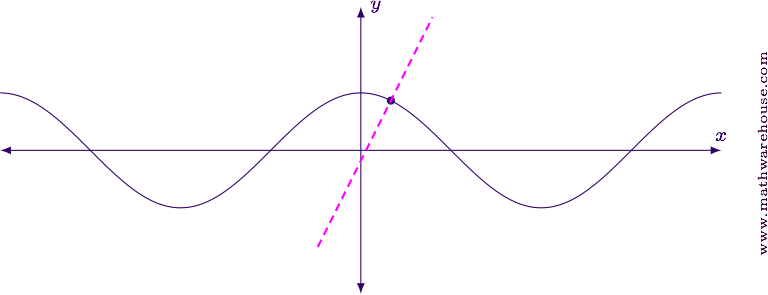
For reference, hither is the graph of the role and the normal line nosotros constitute.

Continue to Practice Problems

Error : Please Click on "Not a robot", and then try downloading once again.

Source: https://www.mathwarehouse.com/calculus/derivatives/how-to-find-equations-of-tangent-lines.php

Posted by: cordonearmeard.blogspot.com

Share this post